Changing Temperature as a Stress to Equilibrium

Changing temperature is the most complex of the changes which can be applied to shift a reaction according to Le Châtelier’s Principle. As such, it will be important to remember that there are two possible reactions - WHAT direction will the system shift and WHY will that shift occur. 

WHAT direction will the reaction shift when temperature is changed?

The effect that an increase (or decrease) of temperature on an equilibrium system depends on whether the (forward) reaction is exothermic or endothermic. If you don’t remember what those terms mean, be sure to review them here.

For this discussion we’ll look at another reaction that chem teachers love:

Co(H2O)6+2(aq) + 4 Cl-1(aq) ⇄ CoCl4-2(aq) + 6 H2O(l)

Once again, teachers favor this reaction to teach Le Châtelier’s Principle because of color. Co(H2O)6+2 is a pink ion while the CoCl4-2 ion is blue. It is, therefore, relatively easy to set up an equilibrium solution that is purple - showing some of both ions. If a stress is applied that shifts the system to the left, the solution gets pinker. If a stress shifts the reaction to the right, the solution gets bluer.

If a nice purple solution is placed in to two separate tests tube and then one is placed in ice water and one is placed in hot water you would see the following:

Clearly, decreasing the temperature shifts the reaction to the left and increasing the temperature shifts the system to the right. To understand this demonstration, you need to know that this particular reaction is endothermic. Since endothermic reactions take in heat when they occur, we can treat heat as a reactant. Let's rewrite that reaction using that idea:

heat + Co(H2O)6+2(aq) + 4 Cl-1(aq) ⇄ CoCl4-2(aq) + 6 H2O(l)

Now we can see that is happening. We know that when we add a reactant, the reaction will shift to the right. So, in this case, if heat is a reactant and we add heat, then the reaction will shift to the right. At the same time if I take heat away (as in the ice beaker above) I am removing a reactant and the reaction shifts to the left.

Here's the problem. NONE of what is written above explains WHY. So, let's tackle that.

WHY does the reaction shift when the temperature is changed?

We have a puzzle we need to unravel. 

1. Two reactions are at the same speed. 

2. BOTH reactions get faster when the temperature increases. 

3. The reaction shifts (which must mean that the change is not the same for both reactions).

The explanation that follows will only make sense if you understand Boltzmann diagrams and how they are affected by temperature, so if you are at all unsure, review that first. You’ll also need to understand energy graphs and how they differ for exothermic and endothermic reactions. Again, if you aren’t sure, review that first.

We will use the endothermic reaction above to work through this problem. Keep in mind that the argument will be essentially the same for exothermic reactions but the “forward” and “backward” situations will be reversed.

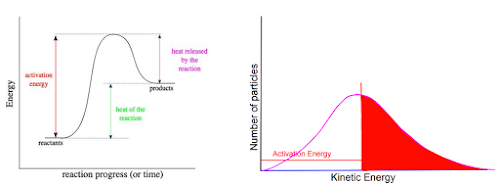
Let’s start by putting an energy graph and a Boltzmann graph next to each other. We’ll use the forward reaction, which is endothermic.

Despite the similar shape, these graphs show very different things

1. The energy graph shows energy required for the reaction

2. The Boltzmann graph shows how much energy molecules have available

3. The energy axis is vertical in the first graph, but horizontal in the second graph

We can relate them through energy though. Not worrying about exact amounts, let’s take the size of the activation energy (vertical on the first graph) and apply that to the horizontal axis on the second graph, like this.

What that means is that ONLY those molecules with at least that much energy can react. To show that better, we'll draw a vertical line and shade the area to the right, like this:

So, in this case, only a small portion of the molecules have enough (or more than enough) energy to react.

Now let's do EXACTLY the same thing at a higher temperature.

Make sure you see what is the same and what is different about these two:

Let’s throw some numbers into the mix. These will be estimates and certainly inaccurate, but they will make the point we need to make.

In the situation on the left (the lower temperature) we could estimate that ~ 20% of molecules have enough energy (or more than enough) to react. At the higher temperature ~50% of the molecules have enough (or more than enough) energy to react.

So, this reaction will go about 2.5 times faster at the higher temperature (50/20 = 2.5).

Now we need to look at the reverse reaction, which for this example is exothermic. That means that the energy graph looks like this. Notice that it has a MUCH smaller activation energy.

When we show that activation energy on a Boltzmann diagram, that will mean that the vertical line that shows "enough" will be much further to the left.

Here are the two graphs at two temperatures, showing a reaction with a lower activation energy.

In the situation on the left (the lower temperature) we could estimate that ~ 80% of molecules have enough energy (or more than enough) to react. At the higher temperature ~90% of the molecules have enough (or more than enough) energy to react.

So, this reaction will go about 1.125 times faster at the higher temperature (90/80 = 1.125).

We can see that BOTH reactions go faster, but the relative change is MUCH larger for the endothermic reaction. That means that, in this case, the backward (exothermic) reaction gets faster, but the forward (endothermic) reaction gets faster-er. So the reaction shifts to the right.

A few important things to keep in mind:

1. Forward and backward are not important. In every reversible reaction (all reactions, really) there is always one direction that is exothermic and one direction that is endothermic.

2. The endothermic reaction is always more affected than the exothermic reaction - that means it gets “faster-er” when the temperature goes up and “slower-er” when the temperature goes down.